Proof.
Fix
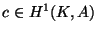
. There is a finite separable extension
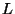
of

such
that
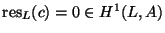
. Let
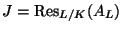
be the
Weil restriction of scalars from
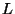
to

of the abelian variety
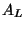
(see [
BLR90, §7.6]).
Thus
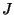
is an abelian variety over

of dimension
![$ [L:K]\cdot \dim(A)$](img51.png)
,
and for any scheme
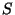
over

, we have a natural (functorial)
group isomorphism
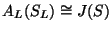
.
The functorial injection
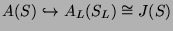
corresponds via Yoneda's Lemma to a natural

-group scheme
map
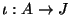
, and by construction
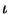
is a monomorphism.
But
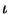
is proper and thus
is a closed immersion (see [
Gro66, §8.11.5]).
Using the Shapiro lemma one finds, after a tedious computation, that
there is a canonical isomorphism
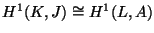
which identifies
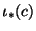
with
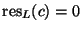
.
