William Stein's homepage



Next: Bibliography
Up: No Title
Previous: Cyclotomic points on modular
The index of an algebraic curve C over
Q is the
order of the cokernel of the degree map
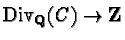 ;
rationality of the canonical divisor implies that the index
divides 2g-2, where g is the genus of C.
When g=1 this is no condition at all; Artin conjectured, and
Lang and Tate [14] proved, that for every integer mthere is a genus one curve of index m over some number field.
Their construction yields genus one curves over
Q only for a few
values of m, and they ask whether one can find genus one curves
over
Q of every index. I have answered
this question for odd m.
;
rationality of the canonical divisor implies that the index
divides 2g-2, where g is the genus of C.
When g=1 this is no condition at all; Artin conjectured, and
Lang and Tate [14] proved, that for every integer mthere is a genus one curve of index m over some number field.
Their construction yields genus one curves over
Q only for a few
values of m, and they ask whether one can find genus one curves
over
Q of every index. I have answered
this question for odd m.
Theorem 8
Let K be any number field. There are genus one curves over K
of every odd index.
The proof involves showing that enough cohomology classes in
Kolyvagin's Euler system of Heegner points do not vanish
combined with explicit Heegner point computations.
I hope to show that curves of every index occur, and
to determine the consequences of my
nonvanishing result for Selmer groups. This can be viewed
as a contribution to the problem of understanding
H1(Q,E).
William A. Stein
1999-12-01