Applications of Taylor Series

Final exam: Wednesday, March 22, 7-10pm in PCYNH 109. Bring ID!
Last Quiz 4: Today (last one)
Today: 11.12 Applications of Taylor Polynomials
Next; Differential Equations

This section is about an example in the theory of relativity. Let $m$ be the (relativistic) mass of an object and $m_0$ be the mass at rest (rest mass) of the object. Let $v$ be the velocity of the object relative to the observer, and let $c$ be the speed of light. These three quantities are related as follows:

$$m = \frac{m_0}{\displaystyle \sqrt{1-\frac{v^2}{c^2}}}$$   (relativistic) mass

The total energy of the object is $mc^2$:

$E = mc^2.$

In relativity we define the kinetic energy to be

$$K = mc^2 - m_0 c^2.$$ (6.4)

What? Isn't the kinetic energy $\frac{1}{2} m_0 v^2$?

Notice that

$$mc^2 - m_0 c^2 = \frac{m_0c^2}{\sqrt{1-\frac{v^2}{c^2}}} - m_0 c^2 = m_0 c^2 \left[ \left(1 - \frac{v^2}{c^2}\right)^{-\frac{1}{2}} - 1\right].$$

Let

$$f\left(x\right) = \left(1 - x\right)^{-\frac{1}{2}} - 1$$

Let's compute the Taylor series of $f$. We have

$$f(x) = (1 - x)^{-\frac{1}{2}} - 1$$

$$f'(x) = \frac{1}{2}(1 - x)^{-\frac{3}{2}}$$

$$f''(x) = \frac{1}{2}\cdot \frac{3}{2} (1 - x)^{-\frac{5}{2}}$$

$$f^{(n)}(x) = \frac{1\cdot 3 \cdot 5 \cdots (2n-1)}{2^n} (1-x)^{-\frac{2n+1}{2}}.$$

Thus

$$f^{(n)}(0) = \frac{1\cdot 3 \cdot 5 \cdots (2n-1)}{2^n}.$$

Hence

$$f(x) = \sum_{n=1}^{\infty} \frac{f^{(n)}(0)}{n!} x^n$$

$$= \sum_{n=1}^{\infty} \frac{1\cdot 3 \cdot 5 \cdots (2n-1)}{2^n \cdot n!} x^n$$

$$= \frac{1}{2}x + \frac{3}{8}x^2 + \frac{5}{16}x^3 + \frac{35}{128}x^4 +\cdots$$

We now use this to analyze the kinetic energy ():

$$mc^2 - m_0 c^2 = m_0 c^2 \cdot f\left(\frac{v^2}{c^2}\right)$$

$$= m_0 c^2 \cdot \left(\frac{1}{2} \cdot \frac{v^2}{c^2} + \frac{3}{8}\cdot \frac{v^2}{c^2} + \cdots\right)$$

$$= \frac{1}{2} m_0 v^2 + m_0 c^2 \cdot \left(\frac{3}{8} \frac{v^2}{c^2} + \cdots\right)$$

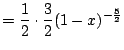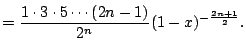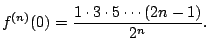
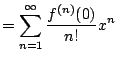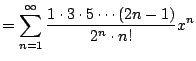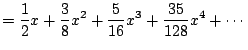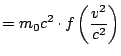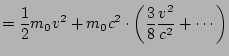And we can ignore the higher order terms if $\frac{v^2}{c^2}$ is small. But how small is ``small'' enough, given that $\frac{v^2}{c^2}$ appears in an infinite sum?