Polar Form

The polar form of a complex number $x+iy$ is $r(\cos(\theta) + i\sin(\theta))$ where $(r,\theta)$ are any choice of polar coordinates that represent the point $(x,y)$ in rectangular coordinates. Recall that you can find the polar form of a point using that

$$r=\sqrt{x^2+y^2}$$    and $$\quad\theta=\tan^{-1}(y/x).$$

NOTE: The ``existence'' of complex numbers wasn't generally accepted until people got used to a geometric interpretation of them.

Example 4.3.2   Find the polar form of $1+i$.
Solution. We have $r=\sqrt{2}$, so

$$1+i = \sqrt{2}\left( \frac{1}{\sqrt{2}} + \frac{i}{\sqrt{2}}\right) = \sqrt{2}\left( \cos(\pi/4) + i \sin(\pi/4) \right).$$

Example 4.3.3   Find the polar form of $\sqrt{3} - i$.
Solution. We have $r=\sqrt{3 + 1} = 2$, so

$$\sqrt{3} - i = 2 \left( \frac{\sqrt{3}}{2} + i\frac{-1}{2} \right) = 2 \left( \cos(-\pi/6) +i \sin(-\pi/6)\right)$$

[[A picture is useful here.]]

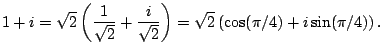
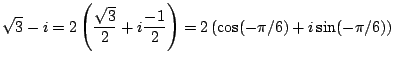
Finding the polar form of a complex number is exactly the same problem as finding polar coordinates of a point in rectangular coordinates. The only hard part is figuring out what $\theta$ is.

If we write complex numbers in rectangular form, their sum is easy to compute:

$$(a+bi) + (c+di) = (a+c) + (b+d)i$$

The beauty of polar coordinates is that if we write two complex numbers in polar form, then their product is very easy to compute:

$$r_1 (\cos(\theta_1) + i \sin(\theta_1)) \cdot r_2 (\cos(\theta_2... ...heta_2)) = (r_1 r_2) (\cos(\theta_1+\theta_2) + i \sin(\theta_1 + \theta_2)).$$

The magnitudes multiply and the angles add. The above formula is true because of the double angle identities for $\sin$ and $\cos$ (and it is how I remember those formulas!).

$$(\cos(\theta_1) + i \sin(\theta_1)) \cdot (\cos(\theta_2) + i \sin(\theta_2))$$

$$= (\cos(\theta_1)\cos(\theta_2) - \sin(\theta_1)\sin(\theta_2)) + i (\sin(\theta_1)\cos(\theta_2) + \cos(\theta_1)\sin(\theta_2)).$$

For example, the power of a singular complex number in polar form is easy to compute; just power the $r$ and multiply the angle.

Theorem 4.3.4 (De Moivre's)   For any integer $n$ we have

$$(r (\cos(\theta) + i\sin(\theta)))^n = r^n (\cos(n \theta) + i\sin(n \theta)).$$

Example 4.3.5   Compute $(1+i)^{2006}$.
Solution. We have

$$(1+i)^{2006} = (\sqrt{2}\left( \cos(\pi/4) + i \sin(\pi/4) \right))^{2006}$$

$$= \sqrt{2}^{2006} \left( \cos(2006 \pi/4) + i \sin(2006 \pi/4) \right))$$

$$= 2^{1003} \left( \cos(3\pi/2) + i \sin(3\pi/2) \right))$$

$$= -2^{1003} i$$

To get $\cos(2006 \pi/4) = \cos(3\pi/2)$ we use that $2006/4 = 501.5$, so by periodicity of cosine, we have

$$\cos(2006 \pi/4) = \cos((501.5)\pi - 250(2\pi)) = \cos(1.5\pi) = \cos(3\pi/2).$$

EXAM 1: Wednesday 7:00-7:50pm in Pepper Canyon 109 (!)
Today: Supplement 1 (get online; also homework online)
Wednesday: Review
Bulletin board, online chat, directory, etc. - see main course website.
Review day - I will prepare no LECTURE; instead I will answer questions.
Your job is to have your most urgent questions ready to go!
Office hours moved: NOT Tue 11-1 (since nobody ever comes then and I'll be at a conference); instead I'll be in my office to answer questions WED 1:30-4pm, and after class on WED too.
Office: AP&M 5111

Quick review:

Given a point $(x,y)$ in the plane, we can also view it as $x+iy$ or in polar form as $r(\cos(\theta) + i\sin(\theta))$. Polar form is great since it's good for multiplication, powering, and for extracting roots:

$$r_1(\cos(\theta_1) + i \sin(\theta_1)) r_2(\cos(\theta_2) + i \si... ...eta_2)) = (r_1 r_2) (\cos(\theta_1 + \theta_2) + i \sin(\theta_1 + \theta_2)).$$

(If you divide, you subtract the angle.) The point is that the polar form works better with multiplication than the rectangular form.

Theorem 4.3.6 (De Moivre's)   For any integer $n$ we have

$$(r (\cos(\theta) + i\sin(\theta)))^n = r^n (\cos(n \theta) + i\sin(n \theta)).$$

Since we know how to raise a complex number in polar form to the $n$th power, we can find all numbers with a given power, hence find the $n$th roots of a complex number.

Proposition 4.3.7 ($n$th roots)   A complex number $z=r(\cos(\theta) + i\sin(\theta))$ has $n$ distinct $n$th roots:

$$r^{1/n}\left(\cos\left(\frac{\theta+2\pi k}{n}\right) + i\sin\left(\frac{\theta+2\pi k}{n}\right) \right),$$

for $k=0,1,\ldots, n-1$. Here $r^{1/n}$ is the real positive $n$-th root of $r$.

As a double-check, note that by De Moivre, each number listed in the proposition has $n$th power equal to $z$.

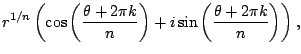
An application of De Moivre is to computing $\sin(n\theta)$ and $\cos(n\theta)$ in terms of $\sin(\theta)$ and $\cos(\theta)$. For example,

$$\cos(3 \theta) + i\sin(3 \theta) = (\cos(\theta) + i\sin(\theta))^3$$

$$=(\cos(\theta)^3 - 3\cos{}(\theta)\sin(\theta)^2) + i(3\cos(\theta)^2\sin(\theta) - \sin(\theta)^3)$$

Equate real and imaginary parts to get formulas for $\cos(3\theta)$ and $\sin(3\theta)$. In the next section we will discuss going in the other direction, i.e., writing powers of $\sin$ and $\cos$ in terms of $\sin$ and cosine.

Example 4.3.8   Find the cube roots of $2$.
Solution. Write $2$ in polar form as

$$2 = 2 (\cos(0) + i \sin(0)).$$

Then the three cube roots of $2$ are

$$2^{1/3} (\cos(2\pi k/3) + i\sin(2\pi k/3)),$$

for $k=0,1,2$. I.e.,

$$2^{1/3}, \quad 2^{1/3}(-1/2 + i \sqrt{3}/2), \quad 2^{1/3}(-1/2 - i \sqrt{3}/2).$$