Definition 1.1 (Projective Space)
Let

be a field and

an integer.
Then
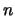
dimensional projective space is, as a set,
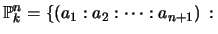
not all $a_i=0$
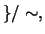
where
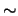
is the equivalence relation in which
for all nonzero
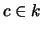
. (Think of
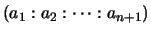
as a ratio.)
Definition 1.2 (Homogeneous Polynomial)
A
homogeneous polynomial is a polynomial
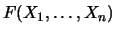
such that
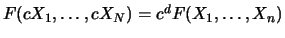
for all
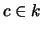
, where
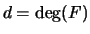
. Equivalently, each of the monomials
in

have the same degree.
Definition 1.3 (Algebraic Variety)
An
algebraic variety in
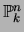
is the set of solutions
to a system
of homogeneous
polynomial equations.
The homogeneity condition ensures that this set is well defined.
Definition 1.4 (Algebraic Plane Curve)
An
algebraic curve in

is the set of solutions
to a single nonconstant homogenous polynomial equation