Two Variables

Consider

$$F(x,y) = 0$$

where $F(x,y)$ is a polynomial in two variables. The graph of $F=0$ is a plane curve.

Let $\deg(F)$ be the largest degree of any monomial that occurs in $F$. E.g., $\deg(x^2y^3 + 2y^4) = 5$.

Simplest case: degree $1$

$$F(x,y) = ax + by + c = 0.$$

The graph is a line.

Since $\deg(P) = 1$, at least one of $a,b\neq 0$. Without loss, suppose that $a\neq 0$. Then

$$x = -\frac{b}{a} y - \frac{c}{a}.$$

and the solutions to $F=0$ are exactly the pairs

$$\left\{\left( -\frac{b}{a} y - \frac{c}{a}, y\right)\, :\, y \in \mathbf{Q}\right\}.$$

Notice that this is an infinite set of solutions.

Next case: degree 2

$$F(x,y) = ax^2 + bxy + cy^2 + dx + ey + f = 0,$$

with $a,b,c,d,e,f\in \mathbf{Q}$.

The graph of $F=0$ is a conic. (Ellipse, hyperbola, ...) (A line usually meets the graph in $2$ points.)

Such an equation may or may not have solutions unlike the linear case (when $F=0$ always has a solution).

Fact: $F=0$ has either no solutions or infinitely many.

Examples

1. $x^2 + y^2 + 1=0$ has no solutions with $x,y\in\mathbf{R}$ (the real numbers), hence no solutions $x,y\in\mathbf{Q}$.
2. $x^2 + y^2 - 1 =0$ (graph is unit circle) has infinitely many solutions. They are
   
   $$\left\{\left(\frac{1-t^2}{1+t^2}, \frac{2t}{1+t^2}\right) \,:\, t \in \mathbf{Q}\right\} \cup \{(-1,0)\}.$$
   
   
   How to find this: Draw the unit circle and a line through $(-1,0)$ with slope $t$. This line is $y=t(x+1)$. Substitute into $x^2+y^2-1$ to find second point of intersection.
   
   $$x^2 + t^2(x+1)^2 - 1 = 0$$
   
   
   
   
   $$(t^2+1)x^2 + 2t^2x + t^2-1=0$$
   
   
   
   
   $$x^2 + \frac{2t^2}{t^2+1}x + \frac{t^2-1}{t^2+1} = 0.$$
   
   
   We know that $x=-1$ is a root, and product of roots is $\frac{t^2-1}{t^2+1}$, so other root is
   
   $$x = \frac{1-t^2}{1+t^2}.$$
   
   
   Thus
   
   $$y=t(x+1) = \frac{2t}{1+t^2}.$$
   
   

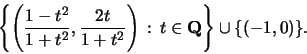
Fact: (mostly explained in Silverman-Tate): When $F(x,y)$ has degree $2$, the equation $F(x,y)=0$ has infinitely many solutions (which we can easily parameterize) if and only if it has at least one solution.

Example:

$$x^2+x^2 = 3$$

has no solution with $x,y\in\mathbf{Q}$.

Proof: $x^2+y^2 = 3$ has a rational solution if and only if $X^2+Y^2=3 Z^2$ has a solution with $X,Y,Z\in\mathbf{Z}$ and $\gcd(X,Y,Z)=1$ (i.e., there is no prime that simultaneously divides all three of $X$, $Y$, $Z$). Suppose that $X,Y,Z$ is such a solution. Then

$$X^2 + Y^2 \equiv 0\pmod{3}$$

so $X^2\equiv Y^2 \equiv 0\pmod{3}$ since the squares modulo $3$ are $0,1$. Thus $3\mid X$ and $3\mid Y$, so $9\mid X^2+Y^2=3Z^2$, so $3\mid Z^2$, hence $3\mid Z$, which contradicts our assumption that $\gcd(X,Y,Z)=1$. DONE.

There is a theory that allows one to decide quickly whether or not a quadratic equation $F(X,Y)=0$ has a solution. I will not discuss it further here, but we can learn more about it in this seminar, if you want.