


Next: Conjectures
Up: Discriminants of Hecke Algebras
Previous: Higher Weight Data
Let  be an even integer and
be an even integer and 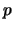 a prime.
Let
a prime.
Let
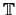 be the Hecke algebra associated to
be the Hecke algebra associated to
 and
let
and
let
 be the normalization of
be the normalization of
 in
in
 .
.
Conjecture 5.1
where
In particular, when
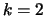
we conjecture that
![$ [\tilde{\mathbb{T}}:\mathbb{T}]$](img132.png)
is not divisible by
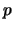
.
Here
 is the binomial coefficient ``
is the binomial coefficient `` choose
choose  '',
and floor and ceiling are as usual. We have checked this conjecture
against significant numerical data. (Will describe here.)
'',
and floor and ceiling are as usual. We have checked this conjecture
against significant numerical data. (Will describe here.)
William A Stein
2002-09-30
![]() be an even integer and
be an even integer and ![]() a prime.
Let
a prime.
Let
![]() be the Hecke algebra associated to
be the Hecke algebra associated to
![]() and
let
and
let
![]() be the normalization of
be the normalization of
![]() in
in
![]() .
.