-
- Aga99b
-
A.Agashe, On invisible elements of the Tate-Shafarevich
group, C. R. Acad. Sci. Paris Sér. I Math. 328 (1999), no. 5,
369-374.
- AS02
-
A.Agashe and W.A. Stein, Visibility of Shafarevich-Tate
groups of abelian varieties, J. Number Theory 97 (2002), no. 1,
171-185.
- AS05
-
A. Agashe and W. Stein, Visible evidence for the Birch and
Swinnerton-Dyer conjecture for modular abelian varieties of analytic rank
zero, Math. Comp. 74 (2005), no. 249, 455-484 (electronic), With
an appendix by J. Cremona and B. Mazur.
- ARS06
-
A. Agashe, K.A. Ribet and W. Stein, The Manin constant,
to appear in Quarterly J. of Pure and Applied Math. volume in honor of J. Coates.
- AM69
-
M.F. Atiyah and I.G.Macdonald,
Introduction to commutative algebra,
Addison-Wesley Publishing Co., Reading, Mass.-London-Don Mills, Ont., (1969).
- BCDT01
-
C. Breuil, B. Conrad, F. Diamond, and R. Taylor, On the modularity of
elliptic curves over
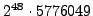 : wild 3-adic exercises, J. Amer. Math. Soc.
14 (2001), no. 4, 843-939 (electronic).
: wild 3-adic exercises, J. Amer. Math. Soc.
14 (2001), no. 4, 843-939 (electronic).
- BCP97
-
W. Bosma, J. Cannon, and C. Playoust, The Magma algebra system. I.
The user language, J. Symbolic Comput. 24 (1997), no. 3-4,
235-265, Computational algebra and number theory (London, 1993).
- CW06
-
M.Ciperiani, A.Wiles, Solvable points on genus one curves,
preprint (2006).
- CFK06
-
C.David, J.Fearnly, H.Kisilevsky,
Vanishing of twisted
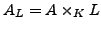 -functions of elliptic curves, to appear in Experiment. Math.
-functions of elliptic curves, to appear in Experiment. Math.
- CM00
-
J.E. Cremona and B. Mazur, Visualizing elements in the
Shafarevich-Tate group, Experiment. Math. 9 (2000), no. 1,
13-28.
- CV92
-
R.F. Coleman, J.F. Voloch,
Companion forms and Kodaira-Spencer theory,
Invent. Math., 110, (1992), 2, 263-281.
- CS01
-
B.Conrad, W.A. Stein,
Component groups of purely toric quotients,
Math. Res. Lett., 8, 5-6, (2001), 745-766.
- Cre
-
J.E. Cremona, Tables of Elliptic Curves,
http://www.maths.nott.ac.uk/personal/jec/ftp/data/
- CR62
-
C.W. Curtis and I. Reiner, Representation theory of finite
groups and associative algebras, Interscience Publishers, a division of John
Wiley & Sons, New York-London, 1962, Pure and Applied Mathematics, Vol. XI.
- Fal86
-
G. Faltings, Finiteness theorems for abelian varieties over number
fields, Arithmetic geometry (Storrs, Conn., 1984), Springer, New York, 1986,
Translated from the German original [Invent. Math. 73 (1983), no. 3,
349-366; ibid. 75 (1984), no. 2, 381] by Edward Shipz, pp. 9-27.
- Ka81
-
N.M. Katz, Galois properties of torsion points on abelian varieties,
Invent. Math. 62 (1981), no. 3, 481-502.
- Kle01
-
T. Klenke, Modular Varieties and Visibility, Ph.D. thesis, Harvard
University (2001).
- KS00
-
D.R. Kohel and W.A. Stein,
Component Groups of Quotients of
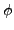 ,
Proc. ANTS-IV, Springer, 2000.
,
Proc. ANTS-IV, Springer, 2000.
- KL89
-
V.A. Kolyvagin and D.Y. Logachev, Finiteness of
the Shafarevich-Tate group and the group of rational
points for some modular abelian varieties, Algebra i Analiz 1
(1989), no. 5, 171-196.
- Maz77
-
B.Mazur, Modular curves and the Eisenstein ideal,
Inst. Hautes Études Sci. Publ. Math., 47, (1977), 33-186.
- Maz99
-
to3em, Visualizing elements of order three in the Shafarevich-Tate
group, Asian J. Math. 3 (1999), no. 1, 221-232, Sir Michael
Atiyah: a great mathematician of the twentieth century.
- Mil72
-
J.S. Milne, On the arithmetic of abelian varieties, Invent.
Math. 17 (1972), 177-190.
- Mil86
-
to3em, Arithmetic duality theorems, Academic Press Inc.,
Boston, Mass., (1986), x+421.
- Rib83
-
K.A. Ribet, Congruence relations between modular forms,
Proc. International Congress of Mathematicians, 503-514, (1983).
- Rib87
-
to3em, On the component groups and the Shimura subgroup of
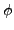 ,
Séminaire de Théorie des Nombres, 1987-1988 (Talence, 1987-1988), Exp. No. 6, 10,
Univ. Bordeaux I.
,
Séminaire de Théorie des Nombres, 1987-1988 (Talence, 1987-1988), Exp. No. 6, 10,
Univ. Bordeaux I.
- Rib90a
-
to3em, On modular representations of
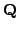 arising from modular forms,
Invent. Math., 100 1990, no. 2, 431-476.
arising from modular forms,
Invent. Math., 100 1990, no. 2, 431-476.
- Rib90b
-
to3em, Raising the levels of modular representations, Séminaire de
Théorie des Nombres, Paris 1987-88, Birkhäuser Boston, Boston, MA, 1990,
pp. 259-271.
- Rib91
-
to3em, Lowering the levels of modular representations without multiplicity one,
International Mathematics Research Notices, (1991), 15-19.
- Rib92
-
to3em, Abelian varieties over
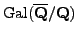 and modular forms, Algebra
and topology 1992 (Taejon), Korea Adv. Inst. Sci. Tech., Taejon, 1992, pp. 53-79.
and modular forms, Algebra
and topology 1992 (Taejon), Korea Adv. Inst. Sci. Tech., Taejon, 1992, pp. 53-79.
- RS01
-
K.A. Ribet and W.A. Stein,
Lectures on Serre's conjectures,
Arithmetic algebraic geometry (Park City, UT, 1999),
IAS/Park City Math. Ser., 9, 143-232, Amer. Math. Soc., Providence, RI, (2001).
- Rub89
-
K.Rubin,
The work of Kolyvagin on the arithmetic of elliptic curves,
Arithmetic of complex manifolds (Erlangen, 1988), 128-136, Springer, Berlin, (1989).
- Se79
-
J-P.Serre, Local fields, Springer-Verlag, New York, (1979).
- Shi94
-
G.Shimura, Introduction to the arithmetic theory of automorphic
functions, reprint of the 1971 original, Kan Memorial Lectures, 1,
Princeton University Press, (1994).
- Ste00
-
W.A. Stein, Explicit approaches to modular abelian varieties,
Ph.D. thesis, University of California, Berkeley (2000).
- Ste04
-
W.A. Stein, Shafarevich-Tate Groups of Nonsquare
Order, Modular Curves and Abelian Varieties, Progress of Mathematics
(2004), 277-289.
- Stu87
-
J.Sturm, On the congruence of modular forms, Number theory (New York, 1984-1985),
Springer, Berlin (1987), 275-280.
- Wil95
-
A.J. Wiles, Modular elliptic curves and Fermat's last theorem,
Ann. of Math. (2), 141(3), (1995), 443-551.
1000
William Stein
2006-06-21